What is Elasticity, Hooke’s Law, Tensile Stress, Strain

In the previous tutorial, we discussed Microscopic and Macroscopic Properties of Matter. In this tutorial discusses the basic theories of elasticity and their applications.

At the end of this tutorial, you will:

• Understand the meaning of elasticity.
• Understand the basic theories behind the elasticity of materials.
• Solve problems in elasticity and
• Apply theories of elasticity in solving real time problems.

What is elasticity?

In physics, elasticity refers to the property of a material that allows it to regain its original shape and size after it has been deformed or subjected to external forces. It is the ability of a material to undergo deformation when a force is applied and return to its original state when the force is removed.

When a material is elastically deformed, the atoms or molecules within the material are displaced from their equilibrium positions but are still held together by interatomic or intermolecular forces. These forces act as springs, pulling the material back to its original shape when the deforming force is released.

Elasticity is often described in terms of stress and strain. Stress is the force applied per unit area, while strain is the ratio of the change in size or shape of a material to its original size or shape.

Hooke’s law

The relationship between stress and strain is described by Hooke’s Law, which states that the stress is directly proportional to the strain within the elastic limit of the material. This linear relationship is expressed as:

Stress = Young’s modulus × Strain

The Young’s modulus is a material property that represents its stiffness or resistance to deformation. Different materials have different Young’s moduli, and it determines how much a material will deform under a given stress.

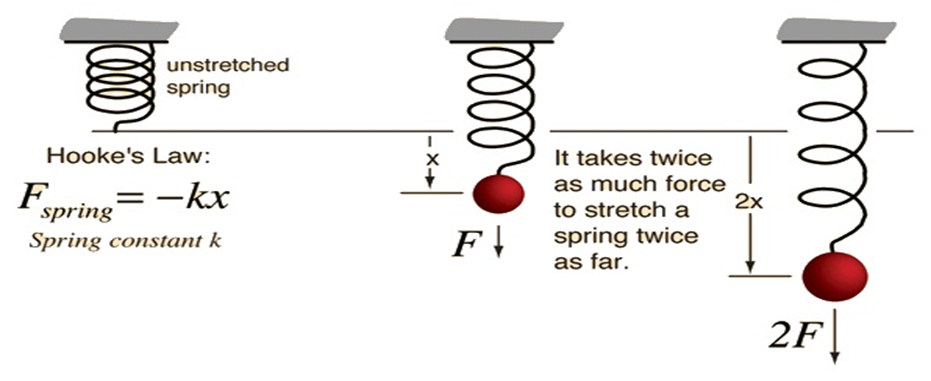
When an elastic material is stretched or compressed, the applied force is given by

\[ F = -k\Delta x \]

Where F is the applied force, Δ is the deformation of the material while k is the force constant. The force constant is a number used to characterize the rigidity of the deforming material. The force constant varies for different materials.

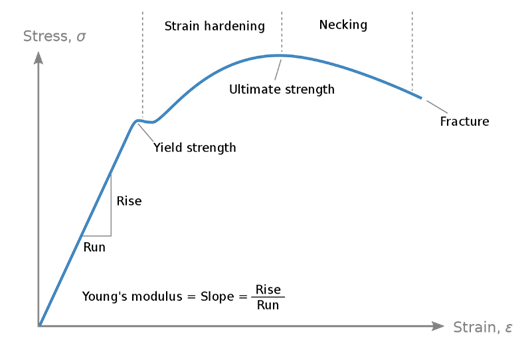
Hooke’s law does have its limits, if a force too great is applied to the elastic material, the elastic limit will be exceeded. After the elastic limit is an area called the plastic region. The yield point begins to deform plastically. Prior to the yield point, the material will deform elastically and will return to its original shape when the applied force is removed. Once the yield point is exceeded, some fraction of the deformation will be permanent and non-reversible. The figure below illustrates the relationships between applied force and deformation. Δ

Elasticity plays a crucial role in many areas of physics and engineering. It is important in understanding the behavior of materials under various conditions, such as the design of structures, the study of mechanical waves, and the analysis of the behavior of solids, liquids, and gases.

Problem 1: A spring has a spring constant of 50 N/m. If a force of 20 N is applied to the spring, what is the resulting deformation?

Solution: According to Hooke’s Law, the force applied (F) is directly proportional to the deformation or displacement (x) of the spring. The equation for Hooke’s Law is:

F = kx

Where F is the force, k is the spring constant, and x is the displacement.

Plugging in the values given:

\[ 20N = (50 \, \text{Nm}^{-1}) \times x \]

Solving for:

\[ x = \frac{{20 \, \text{N}}}{{50 \, \text{N/m}}} = 0.4 \, \text{m} \]

Therefore, the spring will deform by 0.4 meters when a force of 20 N is applied.

Problem 2: A wire has a Young’s modulus of 2.5×〖10〗^10 N⁄m^2 . If the wire undergoes a strain of 0.02, what stress is applied to the wire?

Solution: The relationship between stress (σ), strain (ε), and Young’s modulus (E) is given by:

σ = E × ε

Plugging in the values:

σ = (2.5 × 10^10 N/m^2) × 0.02

σ = 5 × 10^8 N/m^2

Therefore, a stress of 5 × 10^8 N/m^2 is applied to the wire.

These are just a couple of examples of how to solve problems using Hooke’s Law. Remember to use the appropriate units and pay attention to the given values and what is being asked for in the problem.

Stress and Strain

When external forces are applied to objects made of elastic materials, they produce changes in shape and size of the objects. These applied force and change in shape are expressed in terms of stress and strain.

Stress and strain are fundamental concepts that describe the response of materials to external forces and deformations. Let’s delve into each of these concepts in detail:

1. Stress: Stress is a measure of the internal forces within a material that develops in response to external forces. It represents the force per unit area acting on a material. Mathematically, stress (σ) is defined as:

Stress (σ) = Force (F) / Area (A)

Stress is typically expressed in units of force per unit area, such as pascals (Pa) or newtons per square meter (N/m²).

There are different types of stress that can act on a material, including:

• Tensile Stress: It occurs when a material is being pulled apart along its length. The stress tends to elongate the material.
• Compressive Stress: It arises when a material is being squeezed or compressed along its length. The stress tends to compress or shorten the material.
• Shear Stress: It arises when forces act parallel to a surface or when layers of a material slide past one another. The stress tends to deform the material by causing it to shear or distort.

2. Strain: Strain is a measure of the deformation experienced by a material in response to stress. It quantifies how much the material changes in size or shape due to external forces. Mathematically, strain (ε) is defined as:

Strain (ε) = Change in length (ΔL) / Original length (L₀)

Strain is a dimensionless quantity and does not have any units.

Similar to stress, there are different types of strain:

• Tensile Strain: It represents the elongation of a material along its length due to an applied tensile stress.
• Compressive Strain: It denotes the shortening or compression of a material along its length due to an applied compressive stress.
• Shear Strain: It characterizes the distortion or change in shape of a material due to shear stress.

3. Relationship between Stress and Strain: The relationship between stress and strain for a given material is often described by its mechanical properties. One of the most fundamental relationships is expressed by Hooke’s Law, which applies within the elastic limit of a material. Hooke’s Law states that stress is directly proportional to strain:

Stress (σ) = Young’s Modulus (E) × Strain (ε)

The Young’s modulus (E) is a material property that quantifies its stiffness or resistance to deformation. It determines how much a material will deform under a given stress. The higher the Young’s modulus, the stiffer the material.

Beyond the elastic limit, materials may exhibit non-linear or irreversible behavior, leading to plastic deformation or permanent changes in shape.

Stress and strain are crucial concepts in various branches of physics and engineering. They help in understanding the behavior of materials under different conditions, analyzing the strength and integrity of structures, designing resilient materials, and studying phenomena like mechanical waves, elasticity, and material failure.

Here’s an example problem that involves stress and strain:

Problem: A wire with an original length of 2 meters and a diameter of 0.02 meters is subjected to a tensile force of 5000 Newtons. If the wire extends to a final length of 2.04 meters, calculate the stress and strain experienced by the wire.

Solution: To solve this problem, we’ll use the formulas for stress and strain and the given data.

1. Stress: Stress (σ) is defined as the force (F) divided by the cross-sectional area (A) of the wire. The formula for stress is:

Stress (σ) = Force (F) / Area (A)

To find the area, we’ll use the formula for the cross-sectional area of a wire:

Area (A) = π * (diameter/2)^2

Given: Force (F) = 5000 N Diameter = 0.02 m

Calculating the cross-sectional area:

Area (A) = π * (0.02/2)^2 = 0.000314 m²

Now we can calculate the stress:

Stress (σ) = 5000 N / 0.000314 m² = 15,924,171.8 Pa or 15.9 MPa (megapascals)

2. Strain: Strain (ε) is defined as the change in length (ΔL) divided by the original length (L₀) of the wire. The formula for strain is:

Strain (ε) = Change in length (ΔL) / Original length (L₀)

Given: Original length (L₀) = 2 m Change in length (ΔL) = Final length – Original length = 2.04 m – 2 m = 0.04 m

Calculating the strain:

Strain (ε) = 0.04 m / 2 m = 0.02 or 2%

Therefore, the stress experienced by the wire is approximately 15.9 MPa, and the strain is 2%.

Note: In this problem, we assumed that the wire has a uniform cross-section and obeys Hooke’s Law within its elastic limit.

Elastic Moduli

Elastic moduli are parameters that describe the response of materials to applied stress and strain, specifically within the elastic range. Elastic moduli are used to quantify the stiffness or rigidity of materials and determine how they deform under stress. There are three commonly used elastic moduli:

1. Young’s Modulus (E): Young’s modulus, also known as the modulus of elasticity or the elastic modulus, represents a material’s ability to resist deformation under tensile or compressive stress. It quantifies the ratio of stress to strain within the elastic limit of the material. Mathematically, Young’s modulus (E) is defined as:

E = Stress / Strain

\[ \frac{\sigma}{\epsilon} = \frac{\frac{F}{A}}{\frac{\Delta L}{L}} \]

Young’s modulus is specific to a particular material and is typically measured in pascals (Pa) or newtons per square meter (N/m²). It is used to compare the stiffness of different materials. Materials with high Young’s moduli are stiffer and less deformable under stress, while materials with low Young’s moduli are more flexible and easily deformable.

The same relation for Young’s modulus holds for forces in the opposite direction; that is, a strain that tries to shorten an object.

Extension and contraction are opposite types of linear strain. Extension means to get longer while contraction means to get shorter. Whenever a material is extended or contracted by a linear stress in one direction (the x-axis for example), the reverse strain usually takes place in the perpendicular directions (the y and z axes). The directions that are perpendicular to this are called transverse directions.

An axial extension is usually accompanied by a transverse contraction. Stretching a piece of bread makes it get thinner as well as longer. Likewise, an axial contraction is usually accompanied by a transverse extension. Flattening a piece of bread makes it get wider and longer as well as thinner. The ratio of transverse strain to axial strain is known as Poisson’s ratio ().

\[ \nu = -\frac{\frac{\Delta y}{y}}{\frac{\Delta x}{x}} = -\frac{\frac{\Delta z}{z}}{\frac{\Delta x}{x}} = \frac{\epsilon_1}{\epsilon_0} \]

A negative sign is needed to show that the changes are usually of opposite type (+ extension, vs – contraction).

In most common materials, Poisson’s ratio typically ranges between -1.0 and 0.5, where -1.0 represents the theoretical limit of an incompressible material (meaning it does not change its volume when deformed), and 0.5 represents the theoretical limit of a perfectly incompressible and isotropic material (meaning it does not change its shape when deformed).

The sign of Poisson’s ratio is important and indicates the nature of the lateral strain when a material is stretched or compressed:

• If ν is positive (0 < ν < 0.5): The material gets thinner in the lateral direction (compresses) when stretched in the longitudinal direction. This is the most common case for most materials.
• If ν is negative (-1.0 < ν < 0): The material expands laterally when stretched longitudinally. Materials with a negative Poisson’s ratio are known as auxetic materials. These materials are relatively rare, but they have unique properties and are of interest in specific applications.
• If ν is exactly 0.5: The material does not change shape at all when stretched or compressed in one direction. This is only a theoretical limit and is not commonly observed in real materials.

Poisson’s ratio is an important material property used in engineering, especially in fields related to stress analysis, structural mechanics, and materials design. It influences the deformation behavior and stress distribution in structures and components under loading, and its value can affect the overall performance and safety of engineered systems.

2. Shear Modulus (G): The shear modulus, also known as the modulus of rigidity, characterizes a material’s resistance to shear stress. It measures the ratio of shear stress to shear strain within the elastic limit. The shear modulus (G) is given by the formula:

G = Shear Stress / Shear Strain

\[ G = \frac{\frac{F}{A}}{\frac{\Delta x}{l}} = \frac{Fl}{A\Delta x} \]

From the figure:

\[ \frac{\Delta x}{l} = \tan\theta \Rightarrow G = \frac{\frac{F}{A}}{\tan\theta} \]

The shear modulus is used to describe the deformation of materials under shear stress. It is also measured in pascals (Pa) or newtons per square meter (N/m²). Materials with high shear moduli are rigid and exhibit limited shear deformation, while materials with low shear moduli are more prone to shear deformation.

3. Bulk Modulus (K): The bulk modulus represents a material’s resistance to uniform compression or volume changes under hydrostatic pressure. It quantifies the ratio of applied stress to resulting volumetric strain. The bulk modulus (K) is given by the formula:

K = -Volumetric Stress / Volumetric Strain

\[ K = -\frac{\frac{F}{A}}{\frac{\Delta V}{V}} \]

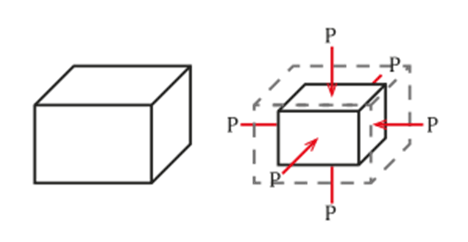
The negative sign indicates that an increase in stress leads to a decrease in volume. The bulk modulus is also measured in pascals (Pa) or newtons per square meter (N/m²). Materials with high bulk moduli are highly resistant to compression and exhibit minimal volume changes under pressure, while materials with low bulk moduli are more compressible.

The inverse of the bulk modulus is called the compressibility.

\[ \kappa = \frac{1}{K} = -\frac{\frac{\Delta V}{V}}{\frac{F}{A}} \]

Elastic moduli play a crucial role in various fields of physics, such as materials science, solid mechanics, and engineering. They help in characterizing the mechanical properties of materials, predicting their behavior under stress and strain, designing structures and components, and understanding phenomena like elasticity, wave propagation, and deformation.

Here are a couple of example problems that involve elastic moduli:

Problem 1: A steel wire with a length of 2 meters and a diameter of 0.01 meters is subjected to a tensile force of 5000 Newtons. If the wire extends by 0.02 meters, calculate its Young’s modulus.

Solution: We can use the formula for Young’s modulus to solve this problem:

Young’s modulus (E) = Stress / Strain

To find the stress and strain, we need to calculate them using the given data:

Given: Original length (L₀) = 2 m Change in length (ΔL) = 0.02 m Force (F) = 5000 N Diameter = 0.01 m

Calculating the stress: The cross-sectional area (A) of the wire can be found using the formula:

Area (A) = π * (diameter/2)^2

Area (A) = π * (0.01/2)^2 = 7.85 x 10^-5 m²

The stress is given by: Stress (σ) = Force (F) / Area (A)

Stress (σ) = 5000 N / 7.85 x 10^-5 m² = 6.369 x 10^7 Pa or 63.69 MPa

Calculating the strain: The strain is given by: Strain (ε) = Change in length (ΔL) / Original length (L₀)

Strain (ε) = 0.02 m / 2 m = 0.01 or 1.0%

Finally, calculating Young’s modulus: Young’s modulus (E) = Stress (σ) / Strain (ε)

Young’s modulus (E) = (6.369 x 10^7 Pa) / 0.01 = 6.369 x 10^9 Pa or 6.369 GPa

Therefore, the Young’s modulus of the steel wire is approximately 6.369 GPa.

Problem 2: A material has a shear stress of 100 Pa when subjected to a shear strain of 0.05. Calculate its shear modulus.

Solution: The shear modulus (G) can be calculated using the formula:

Shear modulus (G) = Shear Stress / Shear Strain

Given: Shear stress (τ) = 100 Pa Shear strain (γ) = 0.05

Using the provided values, we can calculate the shear modulus:

Shear modulus (G) = 100 Pa / 0.05 = 2000 Pa or 2 kPa

Therefore, the shear modulus of the material is 2 kPa.

These are just a couple of examples of how to solve problems involving elastic moduli. Remember to use the appropriate units and apply the relevant formulas based on the given data.